How to Build Correlation Matrices

They key to trend following is to be very diversified, so that your return curve moves up in a reasonably smooth manner. What you need to ask yourself however is whether you are holding a real diversified portfolio or just a very expensive illusion of safety. Everyone talks of increasing correlations the new risk on/risk off world, but few topics are as misunderstood and misused as this one. I’ll try to clear up some of the questions in this tutorial on how to measure and analyze correlations for cross asset futures.

Never Calculate Correlations Based on Prices

The most common rookie mistake is to calculate correlations based on price levels directly. For simple examples it may seem that the numbers make sense and don’t differ much from using returns, but there is an error factor present which may grow significant in some cases. By using absolute price levels as basis for the correlations you are implying that there may be a connection between the price levels themselves across instruments and asses classes, instead of the relative changes in them. As long as the price moves in your series are very evenly distributed you’ll get very close numbers but by using the absolute price levels as your basis, you are putting a very high weight on the earlier numbers in the series and that is probably something you don’t want to do. The oldest return in an N-period data series contributes N times as much to the correlation result as the newest return in the set and that leaves you with nonsense data for our purposes.

Use Log Returns as your Basis

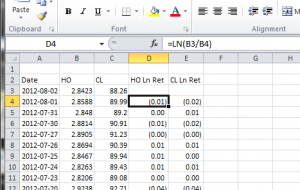
Instead of using absolute price levels, you should use log returns. You could argue that using simple percentage returns gives close enough results, but if you’re going to do it, let’s do it right. The log returns between two consecutive days is simply calculated as ln(P2/P1), where P1 is the price on the first day and P2 the price on the second day. By normalizing your data series this way before making any correlation calculations you’ll get outputs that make a whole lot more sense.

Different Trade Times Can Distort the Picture

If you are only interested in instruments on the same exchange or at least on exchanges with the same closing time, things are so much easier. You simply cannot compare the closing prices for each calendar day across multiple regions of the world, as they represent the closing price of very different times of that day. When the Hang Seng futures close the European futures are just starting trading and the Americans are not even awake yet. A surprise move in the S&P would likely have an impact the next day on the Asian markets, but this would be lost if you use one day return calculations and it would seem as if the moves are not related.

The simple and most common solution is to calculate returns based on several days, effectively diluting this time difference effect. If you for instance calculate the returns as ln(P10/1) and thereby use a ten day return as your basis, you will find that the resulting correlations are much more accurate.

Calculating the Correlations

Correlation calculations are very straight forward and once you have the correct data to base it on, as described above, you can use a normal correlation formula. The one built into Excel works just fine in case you want to work in that environment. What you need to do is to calculate the log returns for all your instruments and then make a big table of correlations between them all. In case you want to read up on the basics of correlations, Wikipedia as usual has an overview.

The correlation number will be between -1 and +1 where the lower extreme means that the series move perfectly opposite, zero that they are completely independent and one if they move perfectly in sync. For our purposes though, it makes little sense to use negative numbers. With trend following futures trading systems, you will never be long two instruments with strong negative correlations or vice versa, that’s just part of how the strategy works.

What we are interested in is primarily to find out where the risk concentrations might be, i.e. which markets have a very high correlation at the moment. If the correlation is negative or positive matters little. In a strong bear market, equities are likely trending down while bonds are trending up. The two asset classes will likely show a high negative correlation. But since we are then with very high probability long bonds and short equities, all that matters is that the correlations are high and not the plus or minus sign. Therefore, it makes sense to only look at absolute correlations.

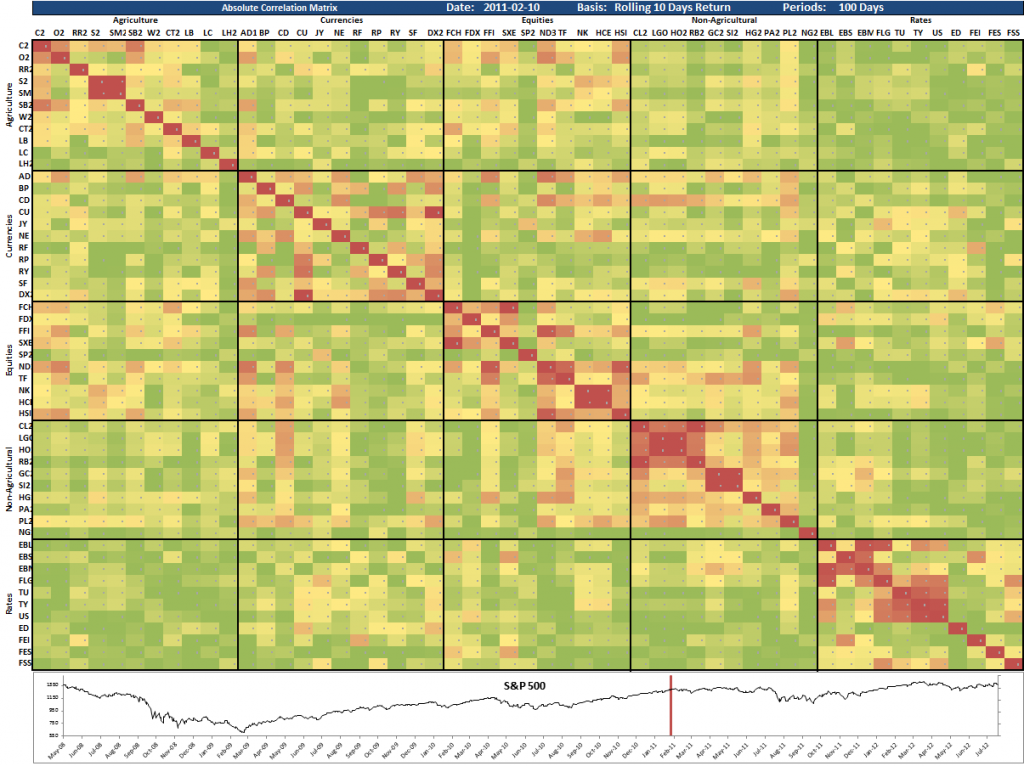
Output Matrix

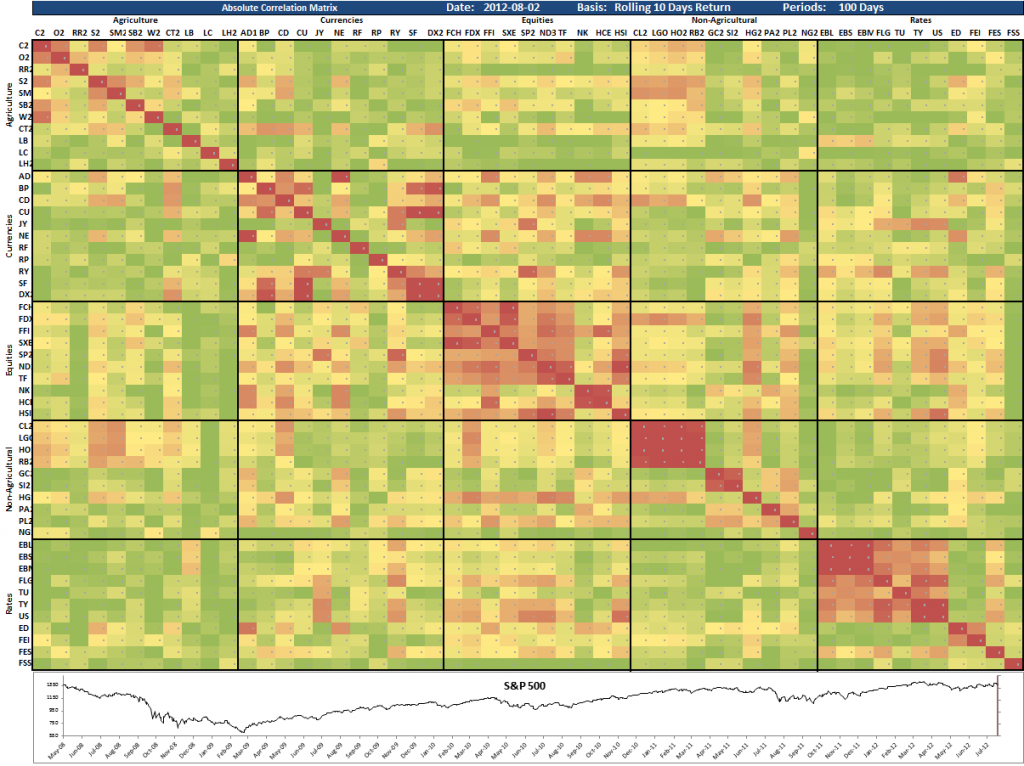
Since we are usually dealing with a large number of instruments when trading futures, it will be easier to read if you group them in a logical manner. In this case, I have grouped the instruments on sectors and made borders around each sector. I’ve also color coded the output and hidden the actual numbers to help get a quick overview of where the concentrations may be. To help get a historical perspective, I’ve also added the S&P 500 index below, showing with a red line where we are at the moment.

The first matrix here is as of August 2, 2012. It tells us that the equity sector has quite a high internal correlation, but this is not something that should surprise anyone. The correlation within this sector is almost always higher than in any other sector and the current level is not historically high.

We can also see that the oil based energy markets have an extremely high correlation at the moment. If you have positions in all of them, you essentially have one big position. The same goes for the bond futures where the correlations are much higher than normal. Be careful if you are heavily long bonds. It may very well continue up, but if it does not you stand to lose on all of them at the same time.

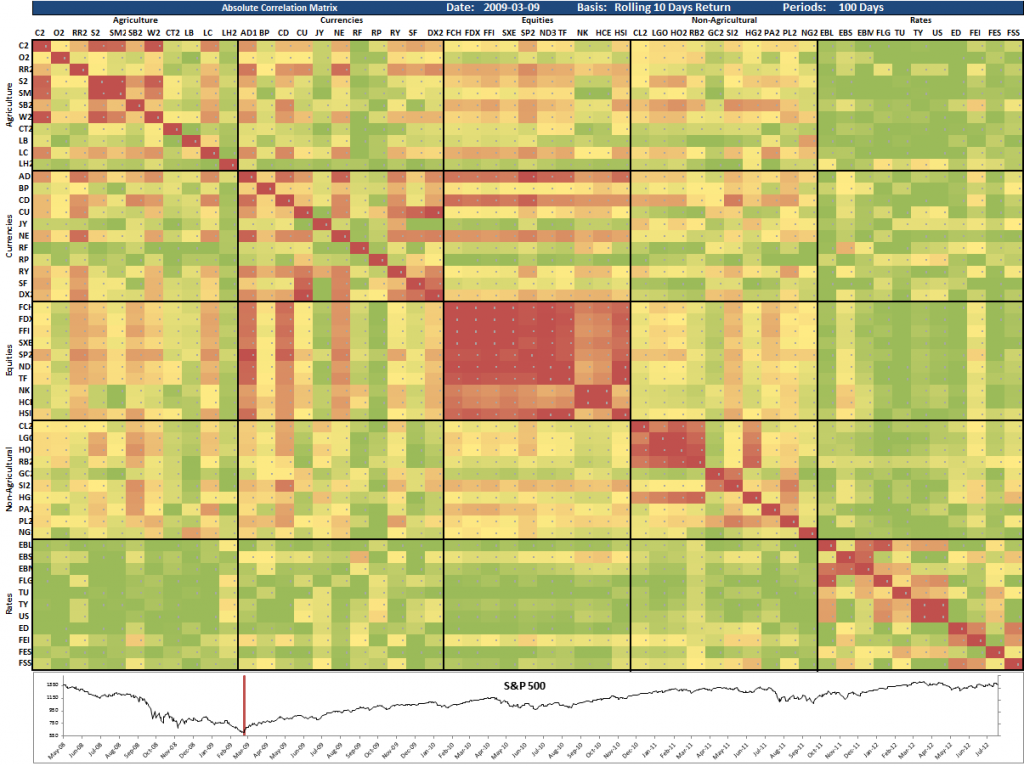
Just to put this matrix into context, let’s show a few older ones to compare with.

 

Happy Correlating!